Template For Standard Deviation Calculator
Example (continued):(9 - 7) 2 = (2) 2 = 4(2 - 7) 2 = (-5) 2 = 25(5 - 7) 2 = (-2) 2 = 4(4 - 7) 2 = (-3) 2 = 9(12 - 7) 2 = (5) 2 = 25(7 - 7) 2 = (0) 2 = 0(8 - 7) 2 = (1) 2 = 1. Etc.And we get these results:4, 25, 4, 9, 25, 0, 1, 16, 4, 16, 0, 9, 25, 4, 9, 9, 4, 1, 4, 9Step 3. Then work out the mean of those squared differences.To work out the mean, add up all the values then divide by how many.First add up all the values from the previous step.But how do we say 'add them all up' in mathematics? We use 'Sigma': Σ.
Imagine you want to know what the whole country thinks. You can't ask millions of people, so instead you ask maybe 1,000 people.There is a nice quote (possibly by Samuel Johnson):'You don't have to eat the whole ox to know that the meat is tough.' This is the essential idea of sampling. To find out information about the population (such as mean and standard deviation), we do not need to look at all members of the population; we only need a sample.But when we take a sample, we lose some accuracy.SummaryThe Population Standard Deviation:The Sample Standard Deviation.

Template For Standard Deviation Calculator That Shows Work
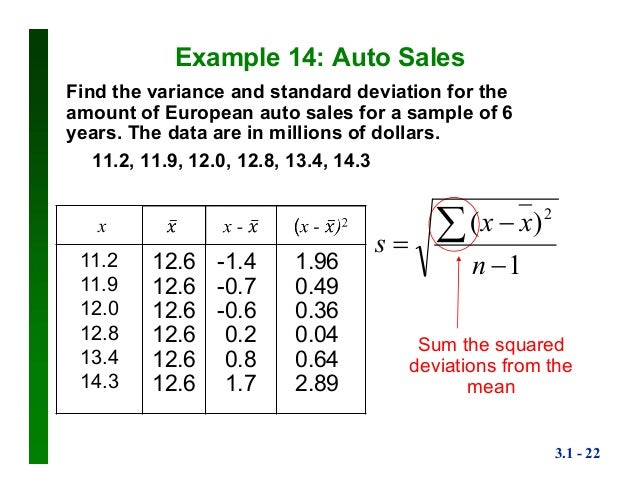
Step 2: Next, determine the number of variables in the sample and it is denoted by n.Step 3: Next, determine the mean of the sample by adding all the random variables and dividing the result by the number of variables in the sample. The sample mean is denoted by x.Step 4: Next, compute the difference between each variable of the sample and the sample mean i.e. X i – x.Step 5: Next, calculate the square of all the deviations i.e. (x i – x) 2.Step 6: Next, add all the of the squared deviations i.e. ∑ (x i – x) 2. Diy mini lazy susans house.